Quantum Information Science and Technology Unit
Professor Kae Nemoto
Abstract
FY2022 was the first year for the unit to operate at OIST, having six researchers and three PhD students joining the unit. During this year, we have started two new national projects: the Moonshot project and the COI-NEXT project, and had a few highlights in our research on quantum error correction, quantum networks, quantum computation and quantum hybrid systems. In particular, we had a breakthrough showing a novel quantum computational model which could solve practical tasks with a number of qubits as small as 10. This new direction will be investigated in more details in the coming years.
1. Staff
- Kae Nemoto, Professor
- Akitada Sakurai, Postdoctoral Scholar
- Henry Nourse, Postdoctoral Scholar
- Hon Wai Lau, Postdoctoral Scholar
- Josephine Dias, Postdoctoral Scholar
- Thomas Scruby, Postdoctoral Scholar
- Nicolo Lo Piparo, Staff Scientist
- Aoi Hayashi, Special Research Student
- Peizhe Li, Special Research Student
- Shin Nishio, Special Research Student
Rotation Students
Ka Wing Yip, Lab Rotation Student (2023 Jan - April)
2. Collaborations
2.1 Architecture and applications for small to large scale quantum computation [MEXT, Quantum Leap Flagship Program] (Principal Investigator: Kae Nemoto)
- Type of collaboration: Joint project
- Researchers:
- Prof. Mio Murao, University of Tokyo
- Dr. William J. Munro and Dr Victor M. Bastidas, NTT
- Prof. Takeaki Uno, National Institute of Informatics
2.2 Large-scale distributed quantum computer architecture [JSPS, Grant-in-Aid for Scientific Research(A)] (Principal Investigator: Kae Nemoto)
- Type of collaboration: Joint research
- Researchers:
- Dr. William J. Munro, NTT
2.3 Non-linear Phenomena in Hybrid Quantum Systems [JSPS, Grant-in-Aid for Scientific Research(A)] (Principal Investigator: William J. Munro)
- Type of collaboration: Joint research
- Researchers:
- Dr. William J. Munro, NTT
2.4 Research and Development of Theory and Software for Fault-tolerant Quantum Computers [Cabinet Office, Moonshot Research and Development Program] (Project Manager: Masato Koashi)
- Type of collaboration: Joint research
- Researchers:
- Dr. Thomas Scruby, OIST
2.5 Scalable and Robust Integrated Quantum Communication System [Cabinet Office, Moonshot Research and Development Program] (Project Manager: Shota Nagayama)
- Type of collaboration: Joint research
- Researchers:
- Prof. David Elkouss, OIST
2.6 Center of Innovation for Sustainable Quantum AI [JST, Program on Open Innovation Platforms for Industry-academia Co-creation (COI-NEXT)] (Project Leader: Shinji Todo)
- Type of collaboration: Joint project
- Researchers:
- Prof. Thomas Busch, OIST
- Dr. Thomás Fogarty, OIST
2.7 Extreme Quantum Information Processing
- Type of collaboration: Joint Research
- Researchers:
- Dr’s. William J. Munro, Koji Azuma and Victor M. Bastidas, NTT
3. Activities and Findings
3.1 Quantum Extreme Reservoir Computation Utilizing Scale-Free Networks
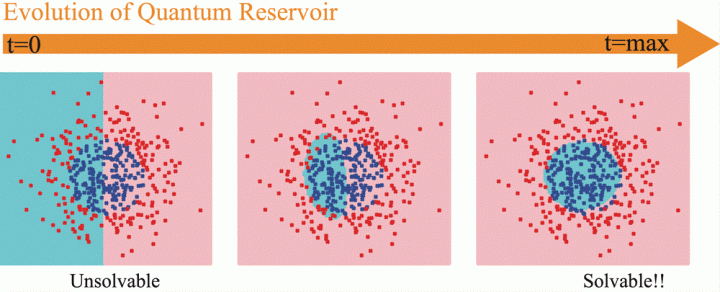
Today’s quantum processors composed of fifty or more qubits have allowed us to enter a computational era where the output results are not easily simulatable on the world’s biggest supercomputers. What we have not seen yet, however, is whether or not the complexity achievable with such quantum processors would ever be useful for any practical applications. In the quantum neural network approach, there has been an expectation that the Hilbert space can serve as a computational resource by providing a large feature space; however, it is not understood, nor illustrated in what way the Hilbert space provides that computational power. In this work we introduced a resource-efficient quantum neural network model with a focus on the role of the feature space the quantum processors can give. Then we applied it for classification tasks, showing a quantum advantage in terms of the physical resources needed to realize the neural network. To facilitate the Hilbert space as a large feature space, we utilize scale-free networks that can be generated in a discrete-time crystal model. The virtue of this approach is in both its theoretical simplicity and its practical applicability: our quantum neural network model is simple enough to identify the role of the Hilbert space as the feature space, and, furthermore, can be implemented with current technology. Our model does not require optimization of the quantum processor, and hence once the quantum processor has been set, it can be used for other different classification problems without any changes in the quantum processor itself.
3.2 Robustness of noisy quantum networks
Quantum networks allow us to harness networked quantum technologies and to develop a quantum internet. But how robust is a quantum network when its links and nodes start failing? We showed that quantum complex networks based on typical noisy quantum-repeater nodes were prone to discontinuous phase transitions with respect to the random loss of operating links and nodes, abruptly compromising the connectivity of the network, and thus significantly limiting the reach of its operation. Furthermore, we determined the critical quantum-repeater efficiency necessary to avoid this catastrophic loss of connectivity as a function of the network topology, the network size, and the distribution of entanglement in the network. From all the network topologies tested, a scale-free network topology showed the best promise for a robust large-scale quantum internet.
3.3 Distributing entanglement in first-generation discrete- and continuous-variable quantum repeaters
Quantum repeaters are used to overcome the exponential photon loss scaling that quantum states acquire as they are transmitted over long distances. While repeaters for discrete-variable encodings of quantum information have existed for some time, approaches for continuous-variable encoding quantum repeaters have only recently been proposed. In this work, we presented a method of using a discrete-variable repeater protocol to distribute continuous-variable states and utilized it to compare the rates of continuous-variable entanglement distribution between first-generation continuous- and discrete-variable quantum repeaters. Such a comparison allowed us to begin benchmarking the two quite different approaches.
3.4 Designing tomorrow’s quantum internet
The principles of quantum mechanics promise a future quantum internet that connects a wide variety of quantum devices together in a coherent and secure fashion. It is well known that due to the size of this quantum internet, quantum repeaters will be a critical part in a similar fashion to the importance of repeaters in today’s telecommunications internet. Given the inherent differences between classical and quantum physics, it is essential to establish how a quantum internet will function including how we route information as well as the functionality quantum repeaters will need to provide. Our considerations went far beyond quantum key distribution and instead focused on a true network of connected quantum devices, including computers and sensors. We showed how the efficient operation of such quantum networks relies on the seamless integration of both quantum and classical communication resources.
3.5 Resource reduction in multiplexed high-dimensional quantum Reed-Solomon codes
Quantum communication technologies will play an important role in quantum information processing in the near future as we network devices together. However, their implementation is still a challenging task due to both loss and gate errors. Quantum error-correcting codes are one important technique to address this issue. In particular, the quantum Reed-Solomon codes well suited to communication tasks as photons can naturally carry more than one qubit of information. The high degree of physical resources required, however, makes such a code difficult to use in practice. A recent technique called quantum multiplexing has been shown to reduce resources by using multiple degrees of freedom of a photon. In this work, we proposed a method to decompose multicontrolled gates using fewer controlled-x (CX) gates via this quantum multiplexing technique. We showed that our method can significantly reduce the required number of CX gates needed in the encoding circuits for the quantum Reed-Solomon code. Our approach is also applicable to many other quantum error-correcting codes and quantum algorithms, including Grovers and quantum walks.
3.6 Non-Pauli errors in the three-dimensional surface code
A powerful feature of stabilizer error correcting codes is the fact that stabilizer measurement projects arbitrary errors to Pauli errors, greatly simplifying the physical error correction process as well as classical simulations of code performance. However, logical non-Clifford operations can map Pauli errors to non-Pauli (Clifford) errors, and while subsequent stabilizer measurements will project the Clifford errors back to Pauli errors the resulting distributions will possess additional correlations that depend on both the nature of the logical operation and the structure of the code. Previous work has studied these effects when applying a transversal T gate to the three-dimensional color code and shown the existence of a nonlocal “linking charge” phenomenon between membranes of intersecting errors. In this paper we generalised these results to the case of a CCZ gate in the three-dimensional surface code and found that many aspects of the problem are much more easily understood in this setting. In particular, the emergence of linking charge is a local effect rather than a nonlocal one. We used the relative simplicity of Clifford errors in this setting to simulate their effect on the performance of a single-shot magic state preparation process and find that their effect on the threshold is largely determined by probability of X errors occurring immediately prior to the application of the gate, after the most recent stabilizer measurement.
3.7 Hardness of Braided Quantum Circuit Optimization in the Surface Code
Large-scale quantum information processing requires the use of quantum error-correcting codes to mitigate the effects of noise in quantum devices. Topological error-correcting codes, such as surface codes, are promising candidates, as they can be implemented using only local interactions in a 2-D array of physical qubits. Procedures, such as defect braiding and lattice surgery, can then be used to realize a fault-tolerant universal set of gates on the logical space of such topological codes. However, error correction also introduces a significant overhead in computation time, the number of physical qubits, and the number of physical gates. While optimizing fault-tolerant circuits to minimize this overhead is critical, the computational complexity of such optimization problems remains unknown. This ambiguity leaves roomfor doubt surrounding the most effective methods for compiling fault-tolerant circuits for a large-scale quantum computer. In this work, we showed that the optimization of a special subset of braided quantum circuits is NP-hard by a polynomial-time reduction of the optimization problem into a specific problem called PlanarRectilinear3SAT.
3.8 InQuIR: Intermediate Representation for Interconnected Quantum Computers
Various physical constraints limit the number of qubits that can be implemented in a single quantum processor, and thus it is necessary to connect multiple quantum processors via quantum interconnects. While several compiler implementations for interconnected quantum computers have been proposed, there is no suitable representation as their compilation target. The lack of such representation impairs the reusability of compiled programs and makes it difficult to reason formally about the complicated behavior of distributed quantum programs. We proposed InQuIR, an intermediate representation that can express communication and computation on distributed quantum systems. InQuIR has formal semantics that allows us to describe precisely the behaviors of distributed quantum programs. We gave examples written in InQuIR to illustrate the problems arising in distributed programs, such as deadlock. We presented a roadmap for static verification using type systems to deal with such a problem. We also provided software tools for InQuIR and evaluated the computational costs of quantum circuits under various conditions.
3.9 Visualizing multiqubit correlations using the Wigner function
Quantum engineering now allows to design and construct multi-qubit states in a range of physical systems. These states are typically quite complex in nature, with disparate, but relevant properties that include both single and multi-qubit coherences and even entanglement. All these properties can be assessed by reconstructing the density matrix of those states—but the large parameter space can mean physical insight of the nature of those states and their coherence can be hard to achieve. Here, we explored how the Wigner function of a multipartite system and its visualization provides rich information on the nature of the state, not only at illustrative level but also at the quantitative level. We tested our tools in a photonic architecture making use of the multiple degrees of freedom of two photons.
4. Publications
4.1 Journals
- Bruno Coelho Coutinho, William John Munro, Kae Nemoto, and Yasser Omar, “Robustness of noisy quantum networks”, Communications Physics 5, 105 (2022), April 28, 2022, https://doi.org/10.1038/s42005-022-00866-7
- Todd Tilma, Mario A. Ciampini, Mark J. Everitt, W. J. Munro, Paolo Mataloni, Kae Nemoto, and Marco Barbieri , “Visualizing multiqubit correlations using the Wigner function”, The European Physical Journal D 76, 90 (2022) (7 pages), May 20,2022, https://doi.org/10.1140/epjd/s10053-022-00419-1
- W. J. Munro, Nicolo' Lo Piparo, Josephine Dias, Michael Hanks, and Kae Nemoto, “Designing tomorrow's quantum internet” AVS Quantum Sci. 4(2), 020503 (2022), June 8, 2022, https://doi.org/10.1116/5.0092069
- Akitada Sakurai, Marta P. Estarellas, William J. Munro, and Kae Nemoto, “Quantum extreme reservoir computation utilizing scale-free networks” Physical Review Applied 17(6), 064044 (2022) (10 pages), June 23, 2022, https://doi.org/10.1103/PhysRevApplied.17.064044
- Thomas R. Scruby, Michael Vasmer, Dan E. Browne, “Non-Pauli Errors in the Three-Dimensional Surface Code” Phys. Rev. Research 4(4), 043052 (2022), October 21, 2022, https://doi.org/10.1103/PhysRevResearch.4.043052
- Josephine Dias, Matthew S. Winnel, William J. Munro, T. C. Ralph, and Kae Nemoto, “Distributing entanglement in first-generation discrete- and continuous-variable quantum repeaters” Phys. Rev. A 106(5), 052604 (2022), November 7, 2022, http://doi.org/10.1103/PhysRevA.106.052604
- Kunihiro Wasa, Shin Nishio, Koki Suetsugu, Michael Hanks, Ashley Stephens, Yu Yokoi, and Kae Nemoto, “Hardness of braided quantum circuit optimization in the surface code” IEEE Transactions on Quantum Engineering 4, 3100207 (pp. 1 -7) (2023), March 2, 2023, https://doi.org/10.1109/TQE.2023.3251358
- Shin Nishio, Nicolò Lo Piparo, Michael Hanks, William John Munro, and Kae Nemoto, “Resource Reduction in Multiplexed High-Dimensional Quantum Reed-Solomon Codes” Phys. Rev. A 107(3), 032620 (2023) (11 pages), March 29, 2023, https://doi.org/10.1103/PhysRevA.107.032620
4.2 Proceedings
- Kunihiro Wasa, Shin Nishio, Koki Suetsugu, Michael Hanks, Ashley Stephens, Yu Yokoi, and Kae Nemoto, “Hardness of braided quantum circuit optimization in the surface code”, Proceedings of QRE2022 (The 4th International Workshop on Quantum Resource Estimation) (2022), June 18, 2022
- Ryo Wakisaka and Shin Nishio, “InQuIR: Intermediate Representation for Interconnected Quantum Computers” Proceedings of QRE2022 (The 4th International Workshop on Quantum Resource Estimation) (2022), June 18, 2022
4.3 Books and other one-time publications
1. 応用物理、第91巻、第8号、p484、 「量子コンピュータがある未来」、根本香絵 (2022)
2. 先端教育(Innovative learning) 「第二次量子革命における日本の教育課題と現状」(2022, November)
4.4 Oral and Poster Presentations
Keynote Presentation
- Kae Nemoto “Quantum computer architecture: from NISQ processors to fault-tolerant quantum computers” Quantum Innovation 2022, Tokyo, Online (November 28, 2022)
Invited Oral Presentation
- Kae Nemoto, “Quantum Internet Stack” Quantum 2.0 Conference and Exhibition, Massachusetts, USA (Tutorial) (June 13, 2022)
- Kae Nemoto, “Quantum computation on scale-free networks in the Hilbert space” QCMC 2022 International Conference on Quantum Communication, Measurement and Computing, Lisbon, Portugal (July 12, 2022)
- Kae Nemoto, “Can NISQ processors be ever useful?” The 1st Conference on Quantum Sciences and Technology, (ConQuest2022), (Virtual conference), Indonesia, (November 22, 2022)
- Josephine Dias “Quantum repeater for continuous-variable entanglement distribution” Quantum Innovation 2022, Tokyo, Online (November 29, 2022)
- Kae Nemoto “Towards the simplest quantum computation “, 24th Australian Institute of Physics Congress, Adelaide, Australia, (2022.12.13)
- Henry L. Nourse “Strong electronic correlations in coordination polymers with density functional theory and slave bosons” JPS 2023 Spring Meeting (session JPS-60071-3), Online, Japan (March 22, 2023)
- Thomas Scruby “Designing Hardware and Software for Linear-Time Quantum Logic” YITP Quantum Error Correction Workshop, Kyoto, Japan (March 22, 2023)
- Thomas Scruby “Local Probabilistic Decoding of a Quantum Code” YITP Quantum Error Correction Workshop, Kyoto, Japan (March 30, 2023)
Oral Presentation
- Shin Nishio “InQuIR: Intermediate Representation for Interconnected Quantum Computers” The 4th International Workshop on Quantum Resource Estimation (QRE2022) co-located with International Symposium on Computer Architecture (ISCA), New York, USA (June 18, 2022)
- Shin Nishio “Bridging the gap between theory and implementation via system software construction for quantum computing” Nano Korea 2022 Satellite Session II IBM Quantum Young Scientist, Gyeonggi-do, Korea (July 8, 2022)
- Shin Nishio “Reducing the resources needed to implement quantum error correction codes using quantum multiplexing” The 15th Pacific Rim Conference on Lasers and Electro-Optics (CLEO Pacific Rim, CLEO-PR 2022), Sapporo, Japan (August 5, 2022)
- Akitada Sakurai “Utilizing quantum dynamics for reservoircomputing” OIST Center for Quantum Technologies Mini Symposium, Okinawa, Japan (November 9, 2022)
- Peizhe Li “Performance of Rotation-Symmetric Bosonic Codes in a Quantum Repeater Scheme based on Cavity-QED” JSPS Japan-Singapore Joint seminar, Tokyo, Japan (February 20, 2023)
- Akitada Sakurai “Utilizing quantum dynamics for reservoircomputing” JSPS Japan-Singapore Joint seminar 2023, Tokyo, Japan (February 21, 2023)
- Aoi Hayashi, “Quantum feature maps in quantum neural networks”, JSPS Japan-Singapore Joint seminar 2023, Tokyo, Japan (February 21, 2023)
- Akitada Sakurai “New dynamical phases on spin-network inspired by DTTS breaking“ CQuERE seminar, Centre for Quantum Engineering, Research and Education, West Bengal, India (February 27, 2023)
- Akitada Sakurai “Utilizing quantum dynamics for reservoircomputing” CQuERE seminar, Centre for Quantum Engineering, Research and Education, West Bengal, India (March 2, 2023)
- Josephine Dias “Reservoir-assisted energy migration in hybrid quantum systems” APS March Meeting 2023, Las Vegas, Nevada (March 8, 2023)
- Josephine Dias “Reservoir-assisted energy migration in hybrid quantum systems” Quantum Materials Seminar, Berkeley, USA (March 14, 2023)
- Peizhe Li “Performance of Rotation-Symmetric Bosonic Codes in a Quantum Repeater Scheme based on Cavity-QED” The 70th JSAP Spring Meeting 2023, Tokyo, Japan (March 15, 2023)
- Aoi Hayashi “量子ニューラルネットワークにおける量子特徴マップの解析” The 70th JSAP Spring Meeting 2023, Tokyo, Japan (March 16, 2023)
Poster Presentations
- Thomas Scruby “Non-Pauli Errors in the Three-Dimensional Surface Code” TQC2022, 17th Conference on the Theory of Quantum Computation, Communication and Cryptography, The University of Illinois at Urbana-Champaign, USA (July 11, 2022)
- Shin Nishio “量子ビット順序変更による Defect Braiding 量子回路最適化の計算量” QEd Summer school 2022 (Quantum Education for Future Technology) , OIST, Okinawa, Japan(September 23, 2022)
- Aoi Hayashi “量子ニューラルネットワークにおける量子特徴マップの解析” QEd Summer school 2022(Quantum Education for Future Technology), OIST, Okinawa, Japan (September 26, 2022)
- Shin Nishio “Defect Braiding 量子回路最適化の計算量的困難性” 第32回量子情報関東スチューデントチャプター研究会, Kanagawa, Japan (October 21, 2022)
- Aoi Hayashi “Quantum feature maps in quantum neural networks” OIST Center for Quantum Technologies Mini Symposium, Okinawa, Japan (November 9, 2022)
- Thomas Scruby “Non-Pauli Errors in the Three-Dimensional Surface Code” 26th Conference on Quantum Information Processing (QIP2023), Ghent, Belgium, (February 6, 2023)
- Shin Nishio, “Computational complexity of optimizing defect braiding quantum circuits by reordering qubits” 26th Conference on Quantum Information Processing (QIP2023), Ghent, Belgium, (February 7, 2023)
- Shin Nishio, “Designing IR for interconnected quantum computers, JSPS Japan-Singapore Joint seminar 2023, Tokyo, Japan (February 20, 2023)
- Henry L. Nourse “Robust, gapped, flat bands at half-filling in the minimal model of the superconducting metal-organic framework, Cu-BHT” APS March Meeting 2023, Nevada, USA (March 9, 2023)
Other Invited Lectures
- Kae Nemoto, “OISTの量子科学技術の取り組み”, 科学技術と経済の会(JATES)「量子技術研究会」, OIST, Okinawa, Japan (November 22, 2022)
- 根本香絵, “量子技術について”, Okinawa Konwakai 33rd General Meeting, OIST, Okinawa, Japan (January 18, 2023)
- Kae Nemoto, ”Quantum dynamics and machine learning”, ML4Q Concepts seminar series (Online), University of Cologne, Germany, University of Cologne, German (February 9, 2023)
- 根本香絵, “パネルディスカッション「量子技術の社会実装への期待」(パネリスト) ", 光・量子飛躍フラッグシッププログラム(Q-LEAP)第5回シンポジウム, Tokyo, Japan (February 28, 2023)
- 根本香絵, “これからの大学の役割とOIST量子技術センターの取組み", 戦略的イノベーション創造プログラム(SIP)第3期課題候補『先進的量子技術基盤の社会課題への応用促進』公開シンポジウム, OIST, Okinawa, Japan (March 23, 2023)
5. Intellectual Property Rights and Other Specific Achievements
Nothing to report
6. Meetings and Events
6.1 OIST Center for Quantum Technologies Mini Symposium
- Date: November 9, 2022
- Venue: OIST B250 Center bldg.
- Organizer: Prof. Kae Nemoto (OIST)
- Invited Speakers
- Akihito Soeda, Associate Professor, Principles of Informatics Research Division, National Institute of Informatics (NII)
- Shohei Watabe, Associate Professor, Shibaura Institute of Technology (SIT)
- Shota Nagayama, Research Associate Professor, Graduate school of Media and Governance, Keio University
- Victor Bastidas, Senior Research Scientist in the Theoretical Quantum Physics Research Group & the Research Center for Theoretical Quantum Physics, NTT BRL.
6.2 Seminars
6.2.1 Theoretical coverage of Homomorphic Encryption and Verifiable Computations and Practical Applications
- Date: October 14, 2022
- Venue: OIST L4F01
- Speaker: Dr. Victor Sucasas and Dr. Eduardo Soria (Technology Innovation Institute)
6.2.2 Cryptography, Cyber Security and Machine Learning: Interdisciplinary benefits
- Date: October 19, 2022
- Venue: OIST C209
- Speaker: Dr. Najwa Aaraj (Technology Innovation Institute)
6.2.3 Universally superposing quantum operations toward quantum functional programming
- Date: December 7, 2022
- Venue: OIST L4F01
- Speaker: Prof. Mio Murao (University of Tokyo)
6.2.4 Critical properties of the Anderson transition in random graphs
- Date: March 8, 2023
- Venue: OIST L4E01
- Speaker: Prof. Gabriel Lemarie (CNRS researcher)
6.2.5 Using superconducting elements to explore the oscillatory Unruh effect and to obtain entangled photon pairs produced from the vacuum
- Date: March 22, 2023
- Venue: OIST C209
- Speaker: Dr. Hui Wang (RIKEN researcher)
7. Other
7.1 OIST Center for Quantum Technologies (OCQT)
The OIST Center for Quantum Technologies (OCQT) has been launched on October 31, 2022.
The OCQT will act as a center for international exchange, helping to facilitate global research collaborations, while attracting and developing current and future leading scientists within the quantum field. The OQT will seek to make contributions to the wide range of quantum technologies, from quantum computers to quantum security, by promoting interdisciplinary research and innovation in quantum physics, computer science, information engineering and other related research fields.
At OIST, we have a strong history in quantum science research. Establishing OCQT, we make a significant step forward towards the realization of quantum technologies with real world impact, as well as deepening our understanding and manipulation of this exotic quantum world. Measurement is the basis of science and technology, and detecting what we could not see before will open up a new technological world previously not accessible. Information processing is now behind all the scientific disciplines. Changing these fundamentally in each field will significantly advance those fields huge as well as create new scientific and technological ones. OCQT strongly supports collaboration across these different scientific disciplines. Our research focuses around but of course is not limited to quantum computation, quantum communication, quantum sensing, quantum cryptography, and cyber security in the quantum era.
https://www.oist.jp/news-center/news/2022/12/11/new-center-quantum-technologies-launched-oist
7.2 External Grants
1.Ministry of Education, Culture, Sports, Science and Technology (MEXT), MEXT - Quantum Leap Flagship Program (MEXT Q-LEAP), Architecture and applications for small to large scale quantum computation (2018-2027) Principal Investigator
2.The Japan Society for the Promotion of Science (JSPS), Grant-in-Aid for Scientific Research(A), Large-scale distributed quantum computer architecture (2021-2025) , Principal Investigator
3.The Japan Society for the Promotion of Science (JSPS), Grant-in-Aid for Scientific Research(A)(PI: William J. Munro), Non-linear Phenomena in Hybrid Quantum Systems (2019-2022), Co-Investigator
4.Cabinet Office, Government of Japan, Moonshot Research and Development Program (Project Manager: Masato Koashi) Research and Development of Theory and Software for Fault-tolerant Quantum Computers (2021-2025), Principal-Investigator
5.Cabinet Office, Government of Japan, Moonshot Research and Development Program (Project Manager: Shota Nagayama), Scalable and Robust Integrated Quantum Communication System (2022-2025), Principal-Investigator
6.Japan Science and Technology Agency(JST), Program on Open Innovation Platforms for Industry-academia Co-creation (COI-NEXT), (Project Leader: Shinji Todo) Center of Innovation for Sustainable Quantum AI (2022-2031), Principal-Investigator