Beyond our physical world: Researcher studies mathematics in other spaces
From designing a new building to figuring out how much time a car drive will take, mathematical formulas are used throughout our world. When equipped with numbers and well-established equations, people of all backgrounds can create processes to aid with productivity and efficiency. But the beauty of mathematics is that it can also leave behind what we can directly observe and move into spaces that are out of reach of reality. To study these rough structures, mathematicians add conditions of their choosing. And the mathematical tools developed from these spaces aren’t just rooted in theory but circle back to the physical world, informing researchers looking at robotics, internet search engines, image classification, and many areas within computer science.
One of these researchers is Professor Xiaodan Zhou from the Okinawa Institute of Science and Technology Graduate University (OIST). Professor Zhou studies functions and partial differential equations in metric spaces and expects to initiate collaborations with fields outside of pure mathematics in the future.
“My interest in mathematics started from a very early age,” said Professor Zhou. “Back then, I loved reading detective novels and murder mysteries. I found that doing mathematical exercises was like solving a puzzle. The way the exercises were designed meant that there must be solution – like a detective working at a crime scene. I first heard about metric spaces during my undergraduate. The whole idea – that we can move into this space where we assume minimal conditions and it still produces a rich theory – was amazing to me.”
Professor Zhou joined OIST in November 2020 to lead the Analysis on Metric Spaces Unit. Originally from China, she worked as a researcher in the United States for several years before moving to Okinawa.
Exploring spaces beyond reality
Over the decades, people have generally found applications for phenomena – such as electricity, advanced technologies, and magnetism – first, with the mathematical foundations being established much later. But, Professor Zhou emphasized, once the reasoning behind the phenomena is realized, then the theory can be improved, the existing applications can be made more efficient and additional applications can be developed.
“This is why it’s really important for mathematicians to continue to explore these phenomena that many people believe we fully understand,” said Professor Zhou.
And, whilst the benefit of studying the physical world may be that we can feel and see what we’re researching, when we move into metric spaces, we can go armed with mathematical tools to understand many properties and functions that cannot be observed directly. “With these tools we can explore phenomena without really experiencing the spaces. This is a really fun and interesting part of mathematics.”
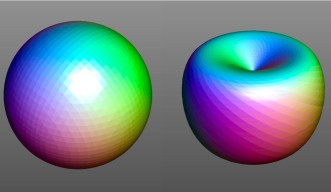
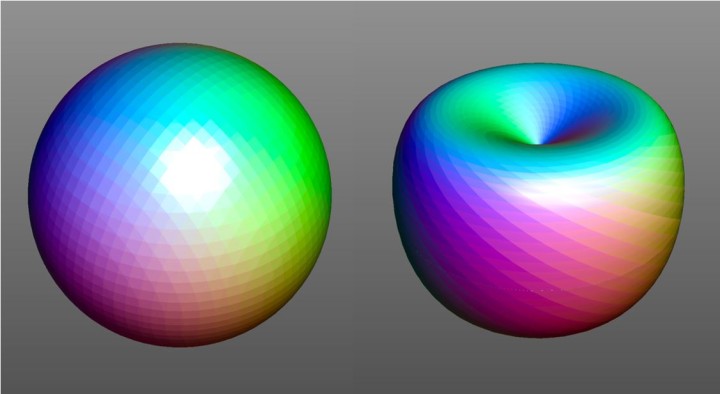
One space that Professor Zhou and her Unit are looking at is called the sub-Riemannian manifolds. Roughly speaking, these spaces are medias where motions are only possible along certain directions and these directions change from point to point. An example of this is a robotic arm that can only move along fixed routes.
Using both analytic and geometric tools the researchers look at these spaces, which are still very similar to classical (or Euclidean) structures but have very different properties and metrics.
“When something can only move in a certain direction, then we look at the path it can take to optimize this restriction,” explained Professor Zhou. “Many well-known questions like the isoperimetric problem, which have been solved and understood well in the Euclidean space, can become very different and complex in this setting. Besides its own mathematical importance, this research is also motivated by the study from many other areas of pure mathematics. Moreover, the study on the sub-Riemannian manifolds has seen numerous applications in engineering and biology including path planning for robotic systems and measuring the geometry of the eye.”
