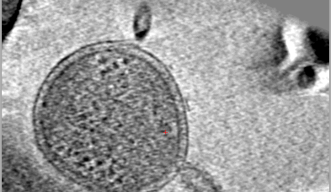
Surviving the Attack of Killer Microbes

The ability to find food and avoid predation dictates whether most organisms live to spread their genes to the next generation or die trying. But for some species of microbe, a unique virus changes the rules of the game. This unusual virus turns some individual microbes into killers. That is, when these killer microbes encounter any other microbe that is competing with them for resources, they kill that microbe on the spot.
One would imagine that eventually, only killer microbes would remain, because all non-killer, or sensitive microbes, would die. But this is not the case. In both wild and in-vitro experiments, the sensitive microbes persist. This question piqued the interest of mathematician Robert Sinclair, a professor at Okinawa Institute of Science and Technology Graduate University who heads the Mathematical Biology unit.
Sinclair first heard about killer microbes from Prof. Alexander Mikheyev in the OIST Ecology and Evolution unit. He credits the design of the university for fostering interactions between researchers. “I would never have known about killer microbes if I had been at a normal university,” explained Sinclair.
Mikheyev, who frequently uses baker’s yeast as his microbial study subject, had been following emerging research on how specific viruses were turning individual yeast cells into killers. “You can look at the question more broadly,” explained Mikheyev, “as ‘What happens when you have different strategies for surviving?’ We cannot see most of the microbial world, and it is run by different laws than our own.”
In the past, researchers have shown that producing more offspring was the sensitive microbes’ key to survival. If the sensitive microbes had a higher reproduction rate, or reproduced far more offspring than the killer microbes, there was no way the killer microbes could kill them all. But no one could assign a number, to show exactly how much more prolific the sensitive microbes would need to be.
As a mathematician, Sinclair saw the question differently: in terms of infinity. “There are many infinities,” said Sinclair, “and they’re all quite different.” In this case, he wondered if only an infinitesimally small change in reproduction rate would make the difference between sensitive microbes’ persistence and death. Instead of modeling hundreds of scenarios, each with a slightly smaller difference in reproduction rate, he would set up a proof and find out.
Sinclair wrote equations somewhat like the logistic growth model, a standard theory that represents population growth. Then he analyzed the equation to determine just how many more offspring the sensitive strain needed to reproduce in order to survive. He found that one doesn't need to measure the reproduction rate between sensitives and killers. As long as the sensitive strain has the higher reproduction rate, it can coexist with killer microbes. His result was published July 14, 2014 in Frontiers in Microbiology.
In addition to solving the killer microbe conundrum, Sinclair’s analysis is unusual because it discusses the infinitesimally small. This idea is typically restricted to pure mathematics, because it is hard to model infinity. “Going to infinity presents trouble,” Sinclair explained. “There are things that can happen that you do not expect.” Applying the infinitesimally small to a real world situation bridges the gap between pure mathematics and applied mathematics. “It’s not that these topics are so monstrously different,” said Sinclair. “I would like to break this artificial boundary pure mathematics and applied mathematics.”
By Poncie Rutsch
Specialty
For press enquiries:
Press Inquiry Form