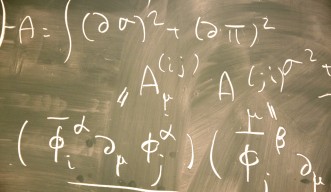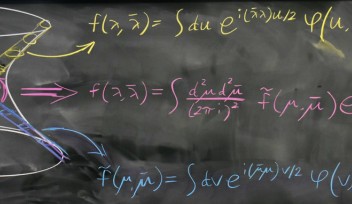The Beauty of Numbers
Mathematics has a knack for describing the universe, so accurately that some contemporary physicists have suggested that reality itself is composed of math. While to some this idea might sound like a confusing nightmare harking back to high school algebra, to others, like Professor Shinobu Hikami, head of the Mathematical and Theoretical Physics Unit at OIST, a universe made of theorems makes for a pleasant daydream. Though only a dream, he says.
“The simplicity of some mathematical proofs is quite beautiful. It’s almost a means of knowing whether your work is sound – if the proof isn’t beautiful, then something is wrong,” says Hikami. “But it’s easy to get consumed by an equation’s charm and place too much emphasis on what mathematics can really do.”
Having spent over 40 years as a professor in mathematics and theoretical physics, Hikami has come across a great deal of phenomena that numbers can explain. Still, he says, there are some things mathematics can’t grasp, and, conversely, ideas in mathematics that have nothing to do with us, or the universe in which we live.
In high school, while his classmates might have been wondering how calculus pertained to everyday life, Hikami was enthralled by the power of numbers, leading him to study different fields of advanced mathematics in his free time. “I had an inspiring mathematics teacher in high school, which really spurred me to become immersed in the subject,” he says. “But at the same time, numbers and equations weren’t enough – I wanted to know how they could be applied.” After taking a physics course as an undergraduate at the University of Tokyo and learning about how math can be used to describe the interactions between all things large and small, he knew he had found his calling.
Hikami has devoted the past 20 years of his research career to random matrix theory – a mathematical technique that finds correlations lurking within large collections of data. Random matrix theory isn’t a run-of-the-mill pair-matching tool – the technique can be used spot clusters of correlated data within a huge set of random numbers, even as the set changes over time, gaining and losing pieces in the process.
In the mid-20th century, Nobelist Eugene Wigner and colleagues first formulated the theory to answer questions in nuclear physics. “When I was student in Tokyo, random matrix theory didn’t receive much attention – only physicists found it intriguing,” says Hikami. But today, the theory’s utility has caught on in fields ranging from economics to biology, though it still remains important in theoretical physics.
An economist might use random matrix theory to see whether the rise and fall of certain stocks are correlated, while a biologist might be interested in finding correlations between the expression level of different proteins in a cell or tissue. In both cases, the theory could be used to confirm correlations between entities that are already known to be connected, like the stocks of car companies and car part manufacturers, or to uncover new and unexpected correlations. While random matrix theory is useful for finding correlations, it can also tell a researcher whether a certain phenomenon is occurring randomly by lacking correlations.
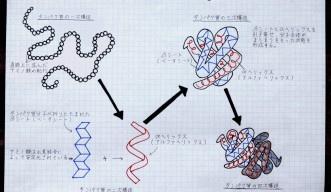
Since human beings, scientists especially, have a tendency to count or measure the world around them, Hikami and other researchers in his field have a wealth of data to investigate for intriguing correlations. When he first started working with random matrix theory, Hikami used the technique to uncover correlations between the vibrations of different strings in string theory. String theory is an area of research in particle physics that claims that elementary particles, such as electrons or photons, aren’t particles at all, but strings that vibrate, which gives them their charge and mass. Working in OIST’s interdisciplinary environment, Hikami has become especially interested in using random matrix theory to uncover correlations in complex biological systems, particularly in the intricate folding and entanglement of amino acid chains – an important process which eventually leads to a three-dimension protein, the building block of body tissue.
“Sometimes solid mathematical theorems lead to theories, which are then verified by experiment. But experimental evidence can also influence a theory to change, and then math does the verifying,” says Hikami. “Either way – in physics, as well as in biology – experiment, math, and theory need to work together to constitute knowledge. And at OIST, we’ve got experimentalists, mathematicians and theorists who do exactly that.”
Specialty
Research Unit
For press enquiries:
Press Inquiry Form