The isoperimetric problem
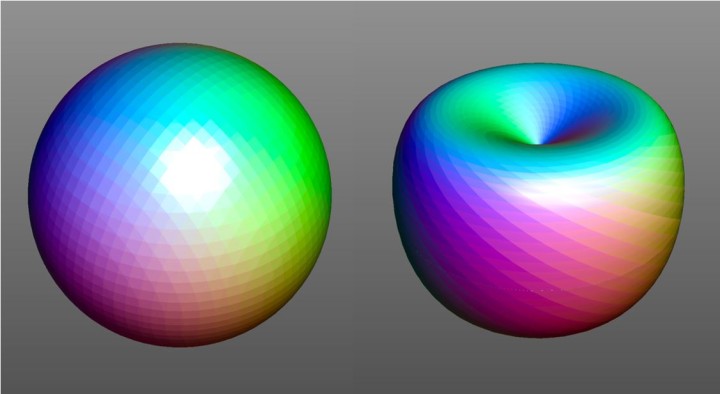
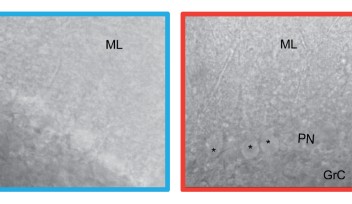
What shape has the minimum surface area that can enclose any given, fixed volume? This is the isoperimetric problem – a common question studied in this branch of theoretical mathematics. In the classical (or Euclidean) space, this shape is a geodesic ball (shown on the left). But move this into the Heisenberg group, a typical sub-Riemannian manifold, with its very different properties and metrics, and the geodesic ball is no longer optimal (shown on the right). In the Heisenberg group, the isoperimetric problem remains open.
What shape has the minimum surface area that can enclose any given, fixed volume? This is the isoperimetric problem – a common question studied in this branch of theoretical mathematics. In the classical (or Euclidean) space, this shape is a geodesic ball (shown on the left). But move this into the Heisenberg group, a typical sub-Riemannian manifold, with its very different properties and metrics, and the geodesic ball is no longer optimal (shown on the right). In the Heisenberg group, the isoperimetric problem remains open.
Copyright OIST (Okinawa Institute of Science and Technology Graduate University, 沖縄科学技術大学院大学). Creative Commons Attribution 4.0 International License (CC BY 4.0).
Tags